What are the relative contributions of the resisting forces of air resistance, weight, rolling resistance & mechanical resistance for long-distance cyclists?

Page Contents:
Types of Resistance
The four types of resistance that prevent a bicycle from endlessly accelerating when pushing on the pedals are:
- Air resistance: Energy lost due to pushing air out of the way.
- Rolling resistance: Energy lost due to the tires deforming as they roll along.
- Gravitational resistance or Weight: Going uphill turns kinetic energy into potential energy. This energy is returned when going downhill, but most of it is lost due to additional air resistance and braking.
- Mechanical resistance: Energy lost due to friction as metal parts rub together, which I divide into resistance in the wheel hubs and efficiency losses in the drivetrain.
Assumed Importance
There has recently been increased interest in air resistance and aerodynamics in cycling as people start to realize that on most routes at typical riding speeds, the aerodynamics of a cyclist is more important than the weight. Aerodynamic road frames and wheels are starting to become common, and so are aero’-road helmets.
Despite this interest, weight is still assumed to be far more important than all other factors if a route involves some significant climbs. The route of the 2016 Transcontinental Race (TCR) provides a good test of this assumption because it included some serious sections of climbing through the Swiss and Italian Alps and the Dinaric Alps in the Balkans. In addition, the predicted riding speed is relatively slow at under 22 kph (see the previous page), and the total weight of the bike and gear (18 kg) is much more than what most cyclists normally ride with. So, in this situation, is weight more important than aerodynamics?
Results & Discussion
See the General Method page for an overview of how the following results were obtained.
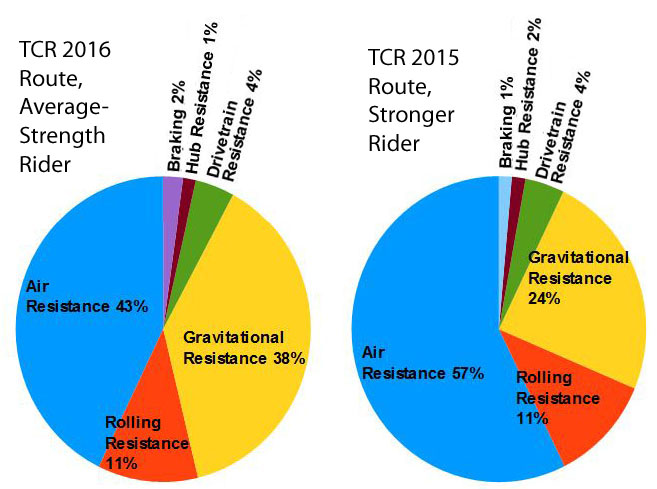
If the relative magnitude of the resisting forces is computed and averaged over the entire 2016 TCR route then, as is shown in the chart above, despite all of the characteristics that should increase the influence of weight (mountainous route, slow speed, and high weight), Air Resistance makes up slightly more of the total resistance (43%) than Weight / Gravitational Forces (38%). On other pages in this section I show that it’s far easier to reduce air resistance significantly (sometimes quite cheaply) than it is to reduce total weight significantly (even when spending a lot of money).
Whereas the previous scenario was likely to be biased towards weight having as large of an effect as possible, the bias can be shifted towards air resistance by looking at the flatter route of the 2015 TCR and looking at the stronger cyclist, who has an average speed of about 26 kph. As is shown in the right-hand chart, air resistance accounted for about 57% of the resistance in this situation compared to only 24% for weight.
After air resistance and weight, the third largest resisting factor is Tire Rolling Resistance, which accounts for 11% of the total resistance in these simulations. Possibly the most surprising result of all of the analyses in this section is that tires are the easiest piece of equipment to change (and one of the least expensive) that can result in substantial amounts of speed/time being gained or lost, possibly affecting finishing times by over half a day based on the tires chosen.
Mechanical Resistance makes up a further 5-6% of all loses, but it could be slightly more if decent components are not used or not cared for. The remaining 1-2% is speed lost due to braking on descents; this factor was included in the model as a simple way to fit the observed speeds on steeper descents better, but as explained on the General Method page, it may not explain exactly what is going on in reality, so this factor is not discussed further.
Last significant page update: November, 2016
This page is in the What Determines Cycling Speed? section. The next page in this section is: